
|
Orbital Confusion
Gola
Paintings such as the often-published one on the left and those depicted on this page, are often described as representing the "gola". This is a Sanskrit word that is usually not translated into Tibetan in texts on astronomy. It basically means circle, and in his short text on gola, Buton Rinchen Drup (bu ston rin chen grub) describes three types of such circle. The first he calls the normal "circle of the world", which is the upper surface of the disks of earth and water that are considered to constitute our world. The surface of the disk of earth beyond the Sīta mountains holds the main twelve continents and the oceans that separate them (for some further details, see here). Beyond lies the great salt ocean that rises up from the lower disk of water. It is these two areas together that Buton refers to as the "circle of the world".The other two circles are essentially the same: the "circle of the (zodiac) signs" and the "circle of the lunar mansions". These refer to the apparent orbit of the Sun, which would be called the ecliptic in modern terms. It is considered in the Kālacakra system that the Sun and the stars of the zodiacal signs and lunar mansions rotate around the central Mt. Meru once every day, bringing the change of day and night to the various continents, and that throughout the year, the Sun moves through the zodiac signs, and a change in orbital disposition brings about the changing of the seasons.
Both of these two main types of gola are depicted in pictures of this kind. The basic gola are described very briefly in the Kālacakra Tantra and its commentary the Vimalaprabhā. Like many such descriptions in this literature, they are so brief as to be open to multiple interpretation, and there are approximately half a dozen different systems described in the Tibetan literature. The most common of these is known as the "Twisted ball of string" (dru gu 'dzings ma).
|
| Single orbital position shown for the "Twisted ball of string". |
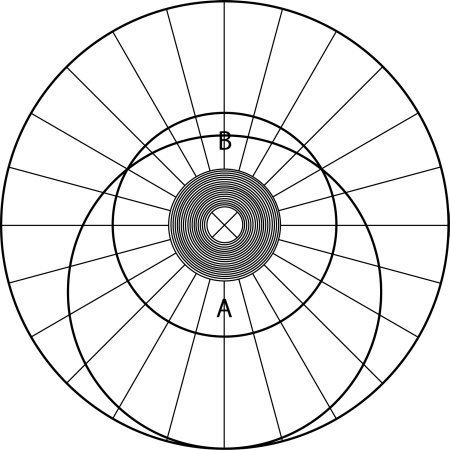
I am yet to see a painting of the "Twisted ball of string" or any other gola system that could be said to be to-scale. Normally the orbit of the Sun is given a smaller diameter, as in the illustration on the right. The small circle in the centre divided into four quadrants represents the base of Mt. Meru. These quadrants would normally be coloured according to the colours of the sides of Meru: east, black (or blue); south, red; west, yellow; and north, white. Usually, one would expect the southern side to be at the bottom – this is where our continent of Jambudvīpa is said to be located – in the position of the letter "A". The tight concentric circles represent the alternating continents, oceans and mountains that are said to surround Meru, there being six of each. The area from the outermost of these tight circles, out to the next circle, which has a diameter that is half of the largest circle, represents Great Jambudvīpa; this is the area that was described above, that holds the main twelve continents, including our own, Jambudvīpa. The area between this circle and the outermost one represents the top surface of the great salt ocean that rises up from the disk of water below.
The circle that is offset and connects with the outer circle at the bottom, and passes through the letter "B", represents the orbit of the Sun on a particular day. If we consider that the letter "A" represents our southern continent of Jambudvīpa, then "B" is the northern continent of Kuru. For any continent, the direction towards the centre, Mt. Meru, is north, and the direction away is south. So, for the continent at "A", the orbit of the Sun is in fact far to the south, as far as it possibly can be. So, for this continent, it is the day of the winter solstice, when the Sun is at its furthest south. However, for continent "B", the orbit of the Sun passes high over head, and the Sun is at its furthest north – the nearest it can get to Mt. Meru. It is therefore the day of the summer solstice in that continent. (Similarly, the eastern and western continents will be experiencing the equinoxes.) If the Sun is right at the bottom of its orbit as depicted here, then it will be mid-day in continent "A", and mid-night in continent "B".
|
| Twelve orbital positions of the "Twisted ball of string". |
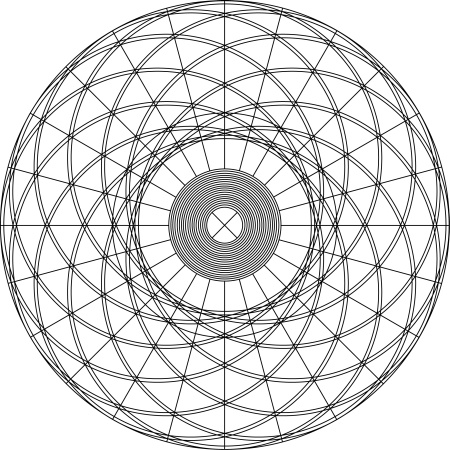
The theory suggests that as well as the Sun travelling around the orbit once a day, the orientation of the orbit itself rotates slowly around Mt. Meru once each year. In this way the continents come to experience the different seasons. Usually, on paintings of this type, twelve positions of the orbit are shown, one each for the entry of the Sun into each of the zodiacal signs. In the illustration on the left these twelve positions are shown, with the orbits being given as bands as described by Mikyo Dorje, but not to the literal scale that he gives in his text.
One further point worth noting here is that it is not only the Sun that is considered to revolve around Meru once each day. The stars, zodiac signs and lunar mansions are all considered to be above the orbit of the Sun, and rotate at almost exactly the same speed, the Sun travelling a little slower, and therefore moving slowly relative to the zodiac signs.
|
| Orbit depicting the supposed shape as described in the tantra. |
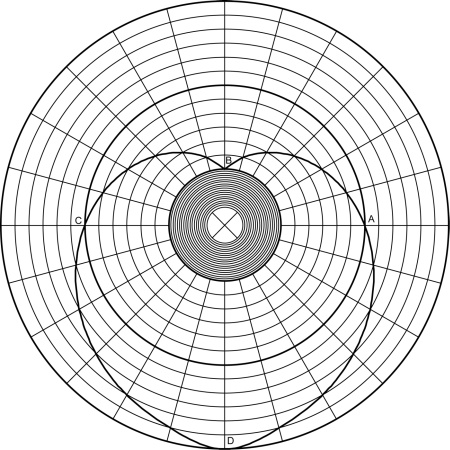
In the Vimalaprabhā commentary, the circle of the lunar mansions and zodiac signs is described in two halves. The northern section, the signs from Aries to Virgo, is said to be shaped like an excellent lotus (petal), and the southern section, the signs from Libra to Pisces, is said to be shaped like a bow. The reason that the first of these two sections is called "northern" is that right in the middle is the beginning of the sign of Cancer, and it is when the Sun enters that sign that it is furthest to the north – this is the time of the summer solstice. Similarly, in the middle of the southern section is the beginning of the sign of Capricorn – the position of the Sun at its most southerly, the time of winter solstice.
But what is the intention of this description of the two halves of the zodiac as having shape? The shape of a bow is commonly meant simply to imply a semi-circle. But the Tibetans normally consider that the shape of a lotus petal is curved, with a sharp inward, or concave, point. This is the interpretation followed by the late Kojo Tsewang Namgyal (Tsenam, see here for a description of his approach to the calendar, developed and published at Sherab Ling monastery, in Himachal Pradesh, northern India.)
A representation of his interpretaion of the gola is shown on the right. The heart-shaped curve represents the orbit of the Sun, and in one sense is more true to the concept of the ecliptic – but more about this later. In Tsenam's system the seasonal points are offset by 23°, but we can ignore this for now. The point "A" is the position of the Sun at the time of the spring equinox, and so this is ususally the First Point of Aries – the point from which longitude is measured. The thick circular line at that point represents effectively the equator. The point "B" is the position of the Sun at its northernmost position, the entry to the sign of Cancer, and the summer solstice. Similarly, the point "C" is the autumn equinox, and point "D" the winter solstice, the entry of the Sun into Capricorn.

|
| Gola drawing by Tsewang Namgyal. |
Now, in Tsenam's system, The Sun does not move around this path once each day, but once each year. The signs of the zodiac stay in their same places relative to the shape of the curve – after all, the curve is said to be the shape of the circle of the ecliptic – the path through wich the Sun travels during the year. Unlike the "Twisted ball of string" system described above, in this interpretation, the whole curve rotates once each day around the centre. This means that if the Sun is at point "B", that point turns in a circle around the centre, remaining the same distance away from the centre at all times. This means that it will be summer solstice for all continents.
This is easily seen in the gola on the left that was made by Tsenam. The orbit itself is made of card, and pinned to the centre of the gola with a yellow pin. Rotate that around fully, and you get the motion of the orbit in one day. This has good and bad points. Certainly it is more true to the nature of the ecliptic, that it itself together with all the stars, Sun and planets, appears to move around the Earth each day. And this is a model that seems to match the Vimalaprabhā description of the shape of the ecliptic. However, this method strongly contradicts the Vimalaprabhā on other points.
The most important of these is that the text describes very clearly that when it is summer in, say, the southern continent, it is winter in the northern continent, and so on for all the others. It describes there as being twelve different northern progressions and twelve different southern progressions. Tsenam's system only allows one. Also, look at the lengths of the signs. Although there are no signs indicated in the diagram above, there are three signs between the points "A" and "B", but also three between "D" and "A". This does not match up with the fact that each sign is considered to contain equal longitude.
In my opinion, a simple error lead to this interpretation being rather compelling. If we look at the diagram above, the thicker circle that passes through the point "A" is equivalent to the equator, and the other circles to lines of latitude on the surface of the Earth. These circles are equally spaced, and just as the distances in a north-south direction over the surface of the Earth between lines of latitude are approximately the same, so the distances in a north-south direction between these lines is also the same. If you project the ecliptic onto the surface of the Earth, it crosses lines of latitude both north and south of the equator. However, there is no linear relationship between longitude along the ecliptic, and latitude of line crossed. The angle at which the ecliptic crosses the equator is greater than the angle that it crosses the next line you choose north, and this continues until you reach the highest point north, where the ecliptic is parallel to a line of latitude – just over 23° for the real Earth.

|
In the diagrams, it is clear that latitude is taken to have a linear relationship to ecliptic longitude, and in some versions of Tsenam's gola, longitude figures are given. If such a linear relationship is accepted, it becomes easy to draw the ecliptic line. The radial lines are all 15° apart, and there is a sufficient number of concentric circles – lines of latitude – to match these. All that is needed next is to connect up successive intersections of these radial and circular lines, starting at the point "B" in the diagram above. The result would seem to many to match the description of the two sections of the circle of the zodiac as given in the Vimalaprabhā – an apparently natural explanation for the enigmatic description of the shape of the zodiac. Mind you, the southern part of this curve, "C"-"D"-"A", is not exactly a semi-circle.
In the close-up on the right, one can see that simple constellations have been drawn around the ecliptic card. At the top right can clearly be seen the Pleiades, and at the bottom left, Ursa Minor. The numbers are longitude, and the figures given for the summer solstice point are 5;1,30 – this is equivalent to 7° Gemini. In the Phugpa system, the summer solstice is considered to appear at that position in Tibet, because that tradition considers that as Tibet is far to the east of India, the solstice will be observed there three weeks before it is observed in India. This is completely wrong, of course, but this is the theory underlying the main Tibetan, or Phugpa, calendar! Tsenam did not share that view, but he also considered the solstice to appear at the same longitude, for reasons that I have not yet fully understood.
On a final point, what could possibly be the meaning of the northern part of the ecliptic being likened to an "excellent lotus petal". In my opinion, the meaning is probably very simple – just look at a lotus flower, below. The southern half of the zodiac is said to be like a bow, simply a semicircle, and this part of the zodiac, when it is all above the horizon and visible, lies in a low arc across the sky from east to west. The northern half, on the other hand, when all above the horizon and visible, rises very high into the sky, almost reaching up to the zenith, certainly when observed from India – just like a fine lotus petal. Maybe...

|
| Lotus growing in Dal Lake, Srinagar, Kashmir. |
Last updated 4 February 2010. E. Henning.
Return to Home Page.